In this section, we will look at how differentiation can be used for a variety of purposes.
The curve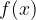 
should be given. At the point 
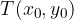we want to determine the tangent to the curve. Since the tangent is a straight line, we start the calculation with the equation for the gradient of a straight line:
From the given form we derive the equation for the tangent to the function 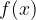at the point :
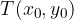The equation of the tangent to the curve 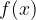at the point 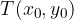is:
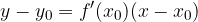
The curve 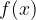should be given. At the point 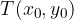, we want to determine the normal to the curve.
We know that a tangent to a curve is a line that touches the curve at a given point. Normal to a curve, however, is a line perpendicular to the tangent and passing through the same point at which the tangent touches the curve. The gradient of the normal is:
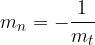
where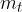 
is the gradient of the tangent.
Calculate the equation of the normal to the function 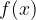at the point :
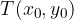The equation of the normal to the curve 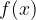at the point 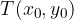is:
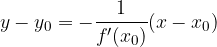
In this subsection, we want to find the angle bounded by a curve and the abscissa (x axis).
A curve and the tangent intersect the abscissa at the same angle:
Therefore, when we calculate the angle between the curve and the x axis, we will calculate the angle between the tangent to the curve and the x axis. We know that the tangent of the inclination angle is equal to the gradient of the tangent:
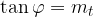
Let's look at how to calculate the angle of inclination:
The tangent of the angle of inclination is equal to the derivative of the function at the point of intersection:
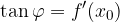
Note: Two angles are complementary when their sum is 90°. Complementarity comes in very handy, as we can understand from the sketch below that the angles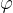 
(angle between curve and x axis) and 
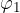(angle between curve and the y-axis) are complementary.
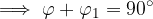
and since we already know how to calculate 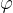(as in the subsection above), we can therefore also calculate 
.
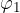The angle between two curves is equal to the angle between the tangents to these two curves at the intersection of the curves. To make it easier to understand, let's look at this graphically:
As between two lines, the angle is calculated as:
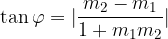
where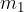 
is the gradient of the first tangent and 
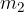is the gradient of the second tangent.
When we sketch the graph of a function, we want to have the sketch as accurately as possible. Therefore, we must determine the increasing and decreasing intervals and the stationary points.
To make it easier to understand the material, let's start with an example that we already know how to solve:
From the example above, we find that the function first decreases, and then begins to increase. We do not know, however, exactly where the turning point is - so here are the typical questions that we do not (yet) know the answers to:
Where (exactly) does the function reach its minimum?
Where (exactly) does the function start to rise and fall?
Does the function have only one minimum or more?
The answers are obtained with the help of differentiation. In doing so, we will need an understanding of local maximum and local minimum when searching for turning points. Local minima and local maxima are called in one word local extremes.
The f function has a local maximum value or local maximum at the point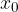 
if all function values on some open interval centered at 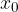are less than the function value 
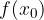. The highest of the local maxima is the global maximum.
The f function has a local minimum value or local minimum at the point 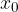if all function values on some open interval centered in 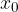are greater than the function value 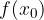. The largest of the local minima is the global minimum.
Note: It may happen that the function at the boundary point of the interval [a, b] has a higher value than the value at the maximum local maximum. Then the function has a global maximum at this boundary point of the interval [a, b]. The same goes for the global minimum.
At points where the derivative of the function is positive, the function increases (the derivative of the function is equal to the gradient of the tangent to the curve at individual points, and if the gradient is positive, the function increases), and at the points where the derivative of the function is negative, the function decreases.
If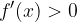 
for each x of the interval (a, b), then the function 
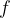on that interval is increasing.
If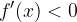 
for each x of the interval (a, b), then the function 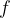on that interval is decreasing.
At the point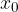 
, where the function passes from decreasing to increasing or vice versa, it has a local extreme. At the point of local extremity, the derivative is equal to 0:
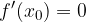
which means that the tangent to the curve has a gradient equal to zero: it is therefore parallel to the abscissa axis, as can be seen from the sketch:
Local extremum point: the gradient (derivative) of the tangent to the curve is equal to 0
The points where the derivative of a function is equal to zero - the tangent is then parallel to the x axis - are called stationary points. There may be a local minimum / maximum or bend at the stationary points.
The derivable function f has a local maximum at point 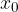if:
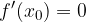
The derivative is positive to the left of 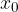and negative to the right of .
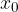The derivable function f has a local minimum at point 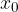if:
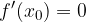
The derivative is negative to the left of 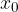and positive to the right of .
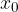However, we also encounter examples of stationary points where the function reaches neither a minimum nor a maximum. These points are called horizontal bends or points of inflexion to which:
The function has a horizontal bend/point of inflexion at the stationary point 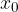, if the derivative does not change its sign in its neighborhood.
Extreme problems are usually problems in which the quantity 1 is determined so that the quantity 2 is maximum / minimum.
