We want to push a load onto a truck. For this purpose, we lean the loading ramp against the truck. A loading ramp is a ramp that helps us get the box onto the truck with less force than if we were to lift it vertically.
A load is pushed onto the truck using an inclined plane
We need to overcome two forces by pushing the load with our hands:
the dynamic component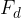 
of the weight of the load; this is the force pushing the load down the plane, and
the frictional force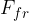 
acting in the opposite direction to the motion of the load as we push, i.e. also down the plane.
How do we calculate the frictional force acting against the motion of the load on the plane? What and how much is the dynamic force? With what force must we push the load up the plane so that it moves uniformly and in a straight line? All these are just some of the questions that we will answer in this material.
A body of mass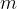 
stands on an inclined plane.
The force of the weight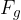 
of the body acts vertically downwards. Let's resolve it into two components:
static component: the first component is perpendicular to the plane. We call it the static component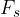 
dynamic component: the second component is parallel to the plane. We call it the dynamic component 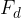. It is also the component of the weight of the body that pushes it down the plane.
Let's see how we calculate them. We make a sketch:
Figure 2: Static and dynamic components of the weight
In Figure 2, we can see we have two similar right-angled triangles:
the first triangle is coloured yellow and has legs with lengths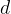 
and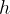 
and a hypotenuse of length 
. It represents the inclined plane itself. In this triangle, 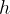is the height and is the length of the plane. The angle between the leg with length 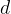and the plane is 
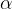. The other angles are shown in Figure 2.
the second triangle is shaded with parallel lines and it represents the triangle of the weight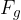 
, static component 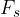, and the dynamic component 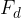of the weight of the load.
The triangles are similar. We can prove this by looking at Figure 2 and determining the congruence of all the angles.
The first way to determine the components of the weight 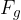of a body on an inclined plane is to write the ratio of the similar sides of the two similar triangles that represent the geometry of the inclined plane and the weight. In Figure 2, two similar sides of both triangles are drawn with the same colour.
Let's first determine the dynamic component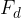 
of the weight of the body using the ratio of the lengths of the similar triangles as shown in Figure 2 above:
Let's also determine the static component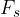 
of the weight of the body using the same procedure:
If we know the angle of inclination of the plane, we can calculate the static and dynamic components of the weight of a body on the plane using trigonometric ratios.
Looking at Figure 2, the static component 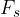can be expressed using the cosine function:
The dynamic component 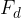can also be expressed using the sine function:
At a given angle 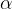, which represents the inclination of the plane with respect to the horizontal, the static component 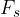and the dynamic component 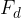of the weight of a body of mass 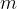are given as:
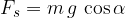
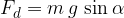
where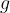 
is the gravitational acceleration.
Let's now observe the forces on a body that is at rest or moving on an inclined plane:
Forces on a body at rest or moving on an inclined plane
In general, the movement of a body on an inclined plane is most often influenced by the following forces:
Dynamic component of the body's weight
The dynamic component of the weight of the body acts through the centre of gravity of the body. This forces the body to move down the plane and its given as:
Frictional force
Another force acting on the body on the slope is:
the static frictional force (if the body is at rest) or
the dynamic frictional force (if the body is moving).
Both forces are calculated by multiplying the coefficient of friction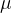 
by the normal force 
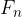perpendicular to the plane. This force is equal and opposite to the static component 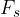of the weight of the body. The frictional force 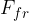acts in the opposite direction as we push the body. The frictional force acts in the opposite direction to the motion of the body.
The static frictional force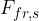 
on the plane is therefore given as:
The dynamic frictional force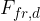 
on the plane is given as:
Traction force
In addition to both forces stated above, the body can also be affected by the traction force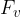 
. This can be directed down or up the plane.
The dynamic component 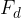of the weight of the body acts downwards along the plane and is given as:
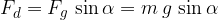
If the body is at rest on the plane, the static frictional force 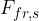acts on it and it's given as:
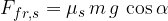
If the body is moving along the plane, the dynamic frictional force 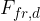acts on it and it's given as:
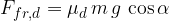
Newton's second law of motion and the Newton's first law of motion also apply on an inclined plane:
A body moves up an inclined plane with uniform acceleration if the sum of all forces acting on it in the direction of the motion is greater than zero.
A body moves uniformly down an inclined plane if the sum of all forces acting on it in the direction of the motion is zero.
