

A cube of mass 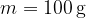 is dropped from the top of a curved smooth surface with radius
is dropped from the top of a curved smooth surface with radius 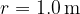 . On its way, the cube slides over a belt of rougher horizontal surfaces with length
. On its way, the cube slides over a belt of rougher horizontal surfaces with length 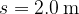 . The coefficient of friction between the rough surface and the cube is
. The coefficient of friction between the rough surface and the cube is 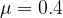 . Then the cube slides over another smooth curved surface on which it stops for a moment and slides back in the opposite direction (see the figure below).
. Then the cube slides over another smooth curved surface on which it stops for a moment and slides back in the opposite direction (see the figure below).
What is the speed at which the cube slides to the rough surface?
What is the velocity of the cube at the end of the rough surface?
By how much does the center of gravity of the cube rise from the end of a rough surface to a position where its velocity is zero.
Where does the cube stop?