A ball is held between two fixed points A and B by means of two stretched springs, as shown in the figure below.
The ball is free to oscillate along the straight line AB. The springs remain stretched and the motion of the ball is simple harmonic. The variation with time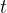 
of the displacement 
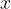of the ball from its equilibrium position is shown in the graph below.
Use the graph above to determine, for the oscillations of the ball,
the amplitude,
the frequency.
Show that the maximum acceleration of the ball is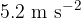 
.
.
.
