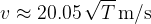Sound is a longitudinal wave of air particles.
As an example, let's take a loudspeaker as a sound source: when the diaphragm moves to the right of the rest position A (see the figure below), a compression of air molecules (higher air density) is created and, as a result, there is an increase in air pressure. As the diaphragm moves to the left, it causes rarefaction (the density of the air decreases) and a decrease in air pressure. The oscillation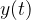 
of the diaphragm overtakes 
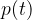by an angle 
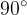, as shown in the figure:
Longitudinal sound velocity
On the receiving side, the process is reversed: increased and decreased air pressure causes the eardrum or microphone diaphragm to oscillate.
A longitudinal wave of air particles is the oscillation of particles in the direction of wave propagation. As a result of longitudinal waves, the air thins and thickens, and the air pressure increases and decreases.
The sound we can hear is in the frequency range of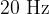 
to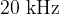 
. Sound with lower frequencies is infrasound, and with higher frequencies is ultrasound.
Compressions and rarefactions spread to the surroundings with the speed of sound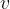 
. In time 
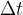, they travel a distance 
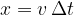, and in the time period 
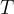of one oscillation, a distance equal to one wavelength 
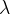of the wave is covered:
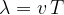
We note that the time period 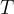is the reciprocal of the frequency 
:
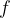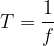
Therefore;
At a given frequency, the wavelength 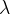will depend only on the speed of sound 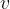. From equation (1), we also express the frequency 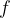as:
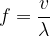
The pressure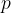 
caused by a travelling sound wave depends on distance 
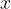and time 
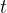. We write this in the form:
Progressive longitudinal wave
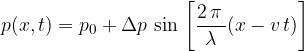
Sound travels not only through air but through any substance that can be compressed under the influence of pressure. The compressibility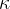 
of the substance is calculated according to the equation:
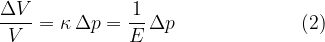
The compressibility 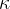of a substance and the modulus of elasticity 
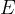are inversely proportional.
We derived the wave speed 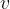in the material, Progressive transverse wave and it is given as:
We note from equation 2 that:
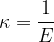
Therefore:
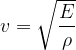
The speed of sound in air is calculated using equation (3) and the gas laws. Let's write down the result without derivation:
Where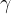 
is the ratio of the specific heat capacity 
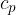at constant pressure to the specific heat capacity 
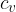at constant volume, this is called adiabatic index. In the case of diatomic molecules, of which air is mainly composed, its value is 1.40.
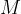is the kilomolar mass of air (
), 
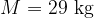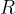is the general gas constant and 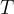is the absolute temperature in Kelvin.
We see that in air, the speed of sound is proportional to the root of the absolute temperature. The proportionality constant is calculated from equation (4) and we get:
