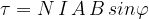Let us imagine a current loop in a magnetic field of a permanent magnet of density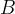 
as shown in the figure below. A magnetic force acts on conductors 
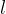, which are perpendicular to the lines of the magnetic field. The distance of this force from the axis of rotation is b/2, i.e. it causes the torque and rotation of the loop.
Torque of a force in a magnetic field
According to the figure, the torque of the two forces is added so that the total torque is:
If the plane of the loop is not in the direction of the magnetic field lines, we take the component of the plane that is oriented in the same way as the magnetic field lines:
The torque on the current loop causes them to rotate (a) to the neutral position (b)
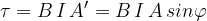
The figure shows a cross-section through the loop. A circle with a cross means that the current goes to the monitor, and a circle with a dot means that it goes from the monitor to the observer.
The vector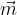 
is called the magnetic moment.
The absolute value of the magnetic moment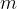 
is the product of the area 
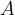of the loop and the current 
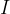flowing through the loop:
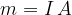
The direction of the magnetic moment is the direction of the magnetic field produced by the loop itself. The angle is the angle between the direction of the magnetic moment and the direction of the field.
The torque is now:
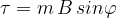
The torque is greatest when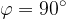 
. The torque twists the loop toward the equilibrium position, where the torque is zero. The direction of the magnetic field forces is then the same as the direction of the magnetic moment - 
.
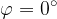If instead of one current loop we have several current loops strung together, we call it a coil. It does not matter whether the cross-section through the coil is a rectangle or a circle. The cross-sectional area 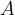, the number of loops 
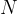, and the angle 
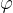between the perpendicular to the coil and the magnetic field direction are important for the magnitude of the torque.
Torque on a coil
The magnetic moment of a loop with 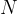turns is 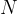times that of one turn:
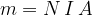
The torque is therefore:
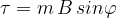
or
