

A weightlifter carries a weight and lifts it first to shoulder height with the force of his hands. He waits a bit, then lifts it above his head with a jerk. Using the strength of his arms and legs, he lifted the weight from the floor to shoulder height. Then to the height of his outstretched arms above his head. The lifter performed work by lifting the weight.
Two physical quantities are required to perform work:
force, and
distance.
It is only when we move a body through a distance using force that work is performed.
When the lifter holds the weight over his shoulders or head, he is not doing any work, even though he has to use the force of his body to overcome the weight of the weight. The weight does not move at this point and since the distance is zero, the work is also zero
Work therefore is the action of force over a particular distance.
We can also work with an electric machine or device. Electrical devices that help us work convert electrical work into mechanical work. This means that we take advantage of the effects of electric current to create the force with which the device can perform work.
In this chapter, we will limit ourselves only to the work done by a force acting parallel to the direction of motion of a body.
Let's assume that a force acts on a body. The body moves and travels due to this force. But we can increase or decrease the speed of an already moving body with force. In both of the above cases, the force does work.
A body moves due to the force applied on it. During the action of the force, the body travels a certain distance. In doing so, we do even more work with:
a greater force, and
a longer distance.
The work is therefore directly proportional to the force applied and the distance travelled.
Work is denoted by 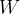 and is given as the product of the applied force and the distance travelled:
and is given as the product of the applied force and the distance travelled:
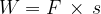
where 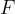 is force, in Newton (N) and
is force, in Newton (N) and  is distance, in meter (m)
is distance, in meter (m)
The unit of work is therefore N m (Newton meter). This unit is also called Joule and is denoted by J:

Work is done only with the force 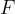 , which acts in the direction of the motion of the body. A force perpendicular to the direction of motion does no work.
, which acts in the direction of the motion of the body. A force perpendicular to the direction of motion does no work.
If we act on a body with force 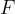 and the body moves a distance
and the body moves a distance  , we have performed work
, we have performed work 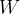 :
:
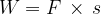
The unit of work is N m (Newton meter) or J (Joule).
The direction of the force relative to the direction of motion of the body can be different. Let's distinguish work:
force acting in the direction of motion of the body,
force acting in the opposite direction to the motion of the body,
force acting at an angle to the direction of motion of the body.
A force can act in the direction of motion of a body (in the direction of the distance). As a result, the body accelerates uniformly. However, if the applied force just overcomes the frictional force, the body moves uniformly. The work of the applied force is positive in both cases since the force and the distance travelled are in the same direction.
If a force acts in the opposite direction to the motion of a body, the body decelerates. Its speed uniformly decreases. In this case, the force does negative work.
However, several forces can act on the body at the same time. For example:
the applied force, and
the braking force (friction or resistance),
If both forces are equal in magnitude and opposite in direction, the body moves uniformly. Such an example is described by Newton's first law. In this case, the applied force does positive work, and the braking force does negative work. Their sum is equal to zero.
Work can be positive or negative. If the force acts in the direction of motion, the work is positive, and if it acts in the opposite direction, the work is negative.
Now, let the force act at an angle to the direction of motion of the body. Only the component of the force that is parallel to the direction of the distance travelled by the body, does work. Therefore, we must resolve the force into two components:
the component parallel to the direction of motion, and
the component perpendicular to the direction of motion.
Work is done only by the component of the force that is parallel to the direction of the distance travelled. The component of the force perpendicular to the motion does no work.
If we apply a force at an angle to the direction of motion of a body, we do work only with the component of the force that is parallel to the direction of motion of the body. A force perpendicular to the direction of motion does no work.
Work and energy are closely related. If we move a body with a force, we say that work is done on the body, and the energy of the body increases. The reverse is also true. If a body has energy, it can do work. Energy is therefore understood as the ability to perform work.
In order to understand this better, let's look at two examples.
Both types of energy, kinetic and potential, are connected to work by the kinetic and potential energy principle.