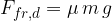In the figure below, a box rests on a flat surface. We apply force on the box to move it. We increase the force until it moves. With the force of our hands, before the box starts moving, we have to first overcome a resisting force called the static frictional force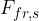 
(left image in Figure 1).
Figure 1: Static frictional force (left) and dynamic frictional force (right)
When the box starts to move, we can slightly reduce the force of our hands (right image in Figure 1). We push the box so that it moves uniformly. According to the law of inertia, the sum of all forces acting on it will then be zero.
We conclude that since the body is moving uniformly, the sum of the forces on the body is zero. Therefore, in addition to our pulling force, another opposing force must act against the pulling force. It is called the dynamic frictional force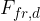 
. This works in the opposite direction to the motion of the body.
The static frictional force is a force that tends to prevent a body from moving. The dynamic frictional force is the force that impedes the movement of a moving body.
Let's now discuss both forces in detail.
A body of mass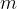 
rests on level ground. It acts on the ground with its weight 
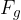, which has a grip in the centre (centre of gravity) of the body and is directed vertically downwards and is given as:
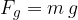
According to Newton's third law, an equally large ground force acts on the body, directed upwards and perpendicular to the ground. Since a perpendicular line is also called a normal, we label the ground force as a normal force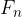 
. It has a grip on the contact between the body and the ground. Therefore:
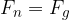
The surface between the body and the ground is rough (see Figure 2):
Figure 2: Body on a rough surface
We first try to move the body with a small force (Figure 3, left). The body does not move; it looks as if it is glued to the surface due to its roughness.
Our small force is opposed by an equally large force, which we call the static frictional force 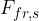. It is directed in the opposite direction to the applied force 
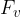. Its grip is on the contact between the body and the surface. The sum of the applied force 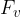and the static frictional force 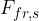is zero and the body is at rest.
Figure 3: The static frictional force resists the traction force
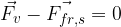
We gradually increase the applied or traction force 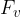. In accordance with the mutual action of forces, the static frictional force 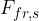also increases. Suddenly, the body shifts and begins to move. Just before the start of the movement, the pulling force 
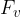and the static frictional force 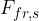are the largest (Figure 3, right).
The static frictional force therefore increases from zero to a particular maximum value as the pulling force increases.
But how do we calculate the maximum static frictional force?
Experiments show that the static frictional force is:
independent of the area of the contact surface;
proportional to the ground or normal force. The proportionality constant depends on the type and roughness of the contact surface and is called the coefficient of static friction,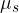 
.
Let's obtain the expression for the static frictional force which is given as:
If a body is located on a flat surface, the maximum static frictional force 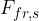depends on the force 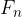exerted by the surface on the body:
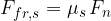
Therefore, for a body on a horizontal base:
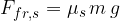
where 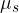is the coefficient of the static friction which depends on the roughness of the contact surface between the body and the ground.
When we overcome the static frictional force and the body starts to move, the dynamic frictional force begins to act on the body (in addition to the traction force) instead of the force of adhesion 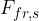. The dynamic frictional force 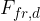opposes the motion of the body.
After we have overcome the static frictional force 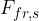, we act on the body with the traction of pulling force 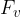. We want to pull the body uniformly and in a straight line, which happens when the pulling force is exactly equal in magnitude and opposite in direction to the dynamic frictional force. We find that we therefore need to reduce the pulling force so that it is slightly less than the force that was needed to overcome the static frictional force (Figure 4, left). From this, we conclude that the dynamic frictional force is a little less than the static frictional force.
Figure 4:Dynamic frictional force
Since the body moves uniformly and in a straight line, according to the law of law of inertia, the sum of all forces acting on it is zero. There is a pulling force 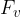and the dynamic frictional force 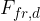acting on the body. Therefore:
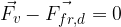
If we increase the traction force 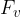, the dynamic frictional force 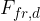will still remain the same. Their difference therefore will no longer be zero and the body will move with uniform acceleration in accordance with the law of dynamics:
If the traction force 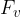falls below the frictional force, the body will stop.
The dynamic frictional force 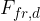is calculated similarly to the static frictional force 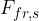. Instead of the static friction coefficient 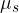, we have the dynamic friction coefficient 
:
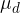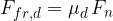
or:
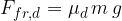
During the motion of the body, we have to overcome the dynamic frictional force 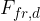. It is given as the product of the coefficient o dynamic friction 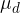and the normal force :
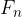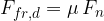
Therefore, for a body on a horizontal base:
