

A characteristic of the motions we learned in the chapters, Uniform Motion for A-LevelUniform motion, Uniformly Accelerated Motion for A-Level, and Vertical Motion under Gravity for A-Levels is that the path of the motion is a straight line. We called it a translational or linear motion.
Also, when the motion consists of two uniform perpendicular motions, the path of the motion is again a straight line - see the chapter, Uniform motion for A-Level. The speed as well as the direction of this motion are the vector sum of the two speeds. The motion is straight and uniform, except that the direction of motion is observed in a plane or in the x-y coordinate system.
If the track path of the motion is a curve (uneven line), it is a curved motion. An example of a curved motion is a projectile motion and a horizontal projectile motion. In both cases, the motion consists of two motions where the velocity vectors are perpendicular to each other and at least one velocity vector changes with time.
The horizontal projectile motion is just a special case of the projectile motion: if the projection motion is a motion where the body has:
an initial velocity 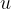 , and
, and
an initial angle at which the body is projected,
the horizontal projectile motion is a motion that has only the initial velocity 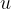 since the initial angle of projection is always 0 degrees (the projection is directed horizontally).
since the initial angle of projection is always 0 degrees (the projection is directed horizontally).
When observing a horizontal projectile motion, the path of the curved motion will be observed in the x-y coordinate system. We will give appropriate indices (x or y) to the velocity vectors.
A body is thrown in a horizontal direction from a height of  as shown in Figure 2 below.
as shown in Figure 2 below.
The body (ignoring the air resistance):
moves uniformly in the horizontal direction and at the same time,
falls at a uniform acceleration in the vertical direction.
Both motions are perpendicular to each other and can be discussed separately. Let’s look at each of the two motions.
The horizontal component of the velocity does not change with time (we assume no air resistance):
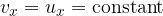
The motion is uniform and in time 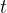 , the body covers a distance
, the body covers a distance 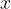 in the horizontal direction which is given as:
in the horizontal direction which is given as:
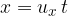
At the time 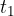 when the body falls to the ground, the motion stops and the body covers a final total distance
when the body falls to the ground, the motion stops and the body covers a final total distance 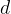 which is given as:
which is given as:
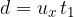
In the vertical direction, the motion is a free fall from a height  with an initial (vertical) velocity of zero. The body moves downward in the negative direction of the y-axis. The final vertical speed is therefore given as:
with an initial (vertical) velocity of zero. The body moves downward in the negative direction of the y-axis. The final vertical speed is therefore given as:
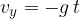
At the same time, its height decreases with time and the height at a time 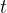 is given as:
is given as:
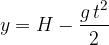
Let's obtain an expression for the time 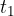 when the body falls to the ground using the formula above:
when the body falls to the ground using the formula above:
Therefore, in a horizontal projectile motion, a body that falls from a height 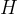 hit the ground in time:
hit the ground in time:
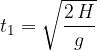
At each moment of the fall, the absolute value of the total (resultant) velocity 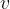 is calculated using Pythagoras' theorem:
is calculated using Pythagoras' theorem:
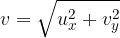
The angle of fall is given as:
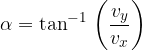
The maximum distance travelled by the body in the horizontal direction is given as:
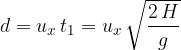
A horizontal projectile motion consists of a horizontal (x) and a vertical (y) component of motion.
Motion in the horizontal direction:
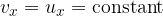
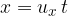
Motion in the vertical direction (initial height is 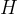 ):
):
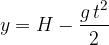
The time taken for the body to fall to the ground is given as:
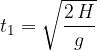
Hence, the horizontal total distance travelled is:
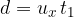
The absolute value of the vector sum of the velocities as a function of time is given as:
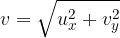
The angle of fall of the body is:
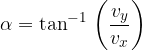
The sum of the two velocity vectors is tangent to the path of the motion of the body at each moment.