A vertical peg is attached to the edge of a horizontal disc of radius 
, as shown in the figure below.
The disc rotates at constant angular speed 
. A horizontal beam of parallel light produces a shadow of the peg on a screen, as shown in the figure below.
(plan view)
At time zero, the peg is at P, producing a shadow on the screen at S. At time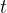 
, the disc has rotated through angle 
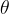. The peg is now at R, producing a shadow at Q.
Determine,
in terms of and 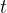, the angle ,
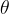in terms of , 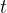and , the distance SQ.
Use your answer to (a)(ii) to show that the shadow on the screen performs simple harmonic motion.
The disc has radius of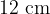 
and is rotating with angular speed of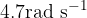 
. Determine, for the shadow on the screen,
the frequency of oscillation,
its maximum speed.
is proportional to the displacement 
. Therefore, the shadow on the screen performs simple harmonic motion.
.
