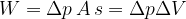When we lift weights, we do work, just like when we drive nails, lift hacks, dig with a shovel, or when we push a car - in all these cases, we do work. There are many more similar examples, all of which are characterized by the fact that we move something by the application of force. We can also find that the bigger the force we apply and the farther we move, the more the work we do. From here, we arrive at the following definition of work:
The work denoted by the letter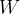 
is defined as the scalar product of the constant force vector 
and the displacement vector 
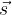of the force.
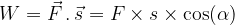
Where 
is the angle between the constant force and the direction of motion.
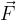is a constant force vector, which means that the magnitude of the force does not change, so we operate with a constant force.
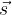is the vector of the displacement of the force, which tells us where and by how much the force has moved.
The unit of work is J (Joule). The work 1 J is the work done when a constant force 1 N moves a body (the point of application of the force) through a distance of 1 m:
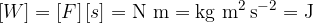
Take, for example, a woman pushing a shopping cart. When she pushes the shopping cart, she does work. If she pushes with a constant force 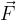in the direction of motion - 
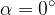- and pushes the cart over a distance 
, the work done by the woman can be calculated using the formula above:
Work done is 
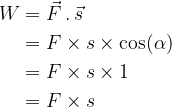
If we also have a child in the shopping cart, the child also pushes the cart with its weight, but perpendicular to the direction of motion -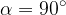 
- and therefore does not do work, because if we calculate the work according to the formula above, we have:
The child does not do work, 
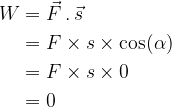
However, when pushing with a constant force at an angle to the direction of motion, we use the formula to calculate the work done:
The work done is 
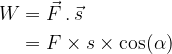
Let us look at the example of rotation shown in the figure below, where a tube wound on a reel is pulled with a constant force 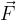. When the reel rotates through an angle 
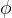, the tube makes a distance 
.
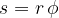In our case, the direction of the force is tangential, which means that the angle between the force and the direction of rotation is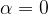 
. Thus, the work can be calculated using the formula:
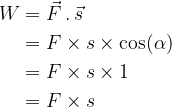
And if we consider that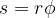 
, we can write:
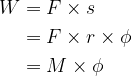
In the end, we wrote the work with torque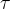 
, since torque is a product of the force 
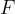and the lever 
:
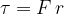
The work 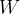of a force in rotation is therefore equal to the product of the torque 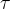of the force and its angular displacement :
The figure shows the pulling of a pipe in the direction of rotation
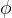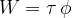
In a case where the force is not in the tangential, i.e., the angle between the force and the direction of rotation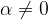 
, only the tangential component 
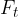of the force is taken into account. Thus we get the formula:
The image shows the pipe being pulled at an angle 
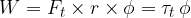
To define the work done by pressure, let's look at a lower piston, which presses on the liquid in a cylinder with a constant force 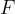and in doing so moves through a distance . The work done is :
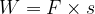
The force 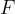can also be expressed in terms of the pressure 
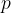as:
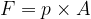
Where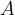 
is the cross-sectional area of the piston (surface of the base surface) and therefore, we have:
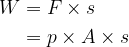
The product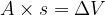 
gives us the volume of the cylinder with the base area 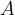and the height , which is equal to the volume of the liquid displaced. Thus we can write:
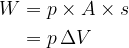
The work done by pressure is therefore equal to the product of the pressure under the piston and the volume of the displaced fluid.
Image shows fluid compression
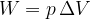
In most cases, we are not really interested in what work the piston did to the liquid but rather, what work was done by the external force pushing the piston.
When we inflate a ball with an air pump, we are not interested in the work done by the piston, but how much work we do in pushing the piston. Therefore, we must take into account that the force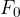 
of air pressure also acts on the outside of the piston, which helps us to push:
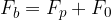
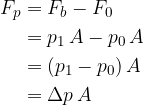
The work of the force with which we push the piston can thus be calculated by the equation:
Image of liquid compression
